What is an inductor?
As a student. I struggled with Maxwell's equations, trying to visualise the interaction of electric and magnetic fields in order to understand what the equations actually meant.
Much later, when I was designing switch-mode power supplies, I came to realise that the key was not the fields themselves, but the energy stored in fields, and what you can do with it ...
Inductance is the property of a component that specifies how big a magnetic field it can generate when a given current flows though it. An inductor is a component whose designer has tried hard to maximise this property.
It's real, like resistance and capacitance, and it can be measured. The size of an inductance is expressed in Henrys (after Joseph Henry, an American contemporary of Faraday). A large choke may have an inductance of 10H or more, whilst that of a small coil may be 100μH or even less. A piece of wire has an inductance of about 25nH per inch (or 1μH/m). There's a more exact formula here .
Current flowing in a wire always causes a magnetic field to appear around the wire. The size and shape and strength of a particular field can in principle be calculated, but the process is difficult and time-consuming. Besides, this information is often not much use to a circuit designer.
The concept of inductance condenses all the complexity of a non-linear magnetic field into a single number. It expresses the geometry of the object causing the field - a wire, or a coil, or a toroid - and also the magnetic properties of the material in the object.
And because inductance is really just a shorthand way of describing the field created by the inductor, it is normally quoted for a given frequency and current level. The inductance might be very different at other frequencies and currents. The 10H choke is designed for 50 Hz mains, and its inductance at 50 kHz (say) would be anybody's guess, but certainly much lower. And at high currents the iron core would saturate. The little coil is intended for use in 100kHz power supplies, and it wouldn't work as well at 1 MHz.
Inductance allows engineers to think in terms of circuits, not fields, which keeps things simpler. But before I get to that, I want to explore the idea of magnetic fields a little further.
What is a magnetic field?
Many definitions say that a magnetic field is a region where magnetic force can be detected. My old school physics book says that IF you could isolate a single magnetic pole (which is impossible), shrink it to a point (you can't), then it would experience a force when placed in the field, and the magnitude and direction of this force (which you couldn't measure) would show the magnitude and direction of the field strength at that point. That's not really helpful at all. It is frustrating to have to imagine what's going on. I live in the real world, and I want to measure what's actually happening.
Some textbooks also prove that magnetic fields aren't 'real'. They are simply the result of charges moving.
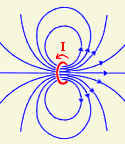
"lines of force" exerted by a current loop
This too is rather disconcerting. Most of us first came across magnetic fields when we began to play with magnets. Magnets are certainly real, and so are the forces they exert. But most people just accept the idea of a magnetic field without worrying about its deeper physical meaning. A magnetic field (like an electric field) provides a convenient shorthand way of thinking about real events, just as young children happily accept that Santa Claus brought their Christmas presents. The toys give just as much pleasure whether they come from Santa Claus or by a more complex route.
Magnetic fields are usually described in terms of a "flux" (whatever that may be) which takes the form of "lines of force", shooting out from one end of a magnet like fireworks and then magically curving round to dive back into the other end. Strong magnets produce more "lines" than weaker ones, which leads to the idea of flux density - the amount of flux passing through a given area. In essence, more flux means more force. Modern loudspeakers use extremely powerful magnets, which makes them small, and linear, and far too loud.
Magnetic fields
It's easy to visualise charge carriers trundling along a wire like a train on a track. Electric and magnetic fields are a more abstract idea. It's harder to visualise fields, even though they are just as common. Every wire carrying a current has a magnetic field surrounding it, and caused by it. The problem is, a wire is nearly one-dimensional, and a field is emphatically three-dimensional.
The magnetic field around a wire is like a tornado (with the current flowing vertically through the tornado's axis). The magnetic field extends outwards at right-angles to the wire, but the force exerted by the magnetic field is at right-angles to that. In other words, the direction of the force is around the axis, much as the debris in a tornado swirls in circles, parallel to the ground.
Unlike electrostatics - the study of charges - where Coulomb's law pretty much explains everything, field theory has many laws to describe the relationship between a current-carrying wire and the magetic field around it. For example, there's the Biot-Savart law, which relates the density of the magnetic field at a point near a wire, and Ampere's law, which does much the same for the field's intensity, and Faraday's law, and Lenz's law, and so on. To describe a field properly demands proper mathematics. Vector algebra is not something most people find easy, with its dot-products and cross-products, not to mention the div, grad and curl that make Maxwell's equations so deceptively simple. There's plenty of this stuff in the textbooks, and no need for more of it here.
I think of a field as a region of distorted space in which things behave in unexpected ways. For example, a leaf floating gently down a stream will be suddenly and unexpectedly accelerated if it happens to drift past a submerged rock. The uniform 'field' of the stream is disturbed by the presence of the rock. Similarly, current flowing in a wire disturbs a nearby compass needle. Fields 'explain' action-at-a-distance - how can a magnet push another one away without touching it? Or at least, they provide a way of thinking about the problem. Fields might be invisible, but their effects are not. It's only the dust and rubbish caught up by a tornado that makes it visible, but the forces it exerts are real, and dangerous.
Of course, we live inside the earth's own magnetic field. A compass points to magnetic north, somewhere near the north pole. If I magnetise a needle, by stroking it several times over the pole of a powerful magnet, and float it on water (and this is easier than it sounds - surface tension is quite strong enough to support it), it points north. If then the magnetic field around the magnet is disturbed by passing a current though a nearby wire, the needle swings to line up with the direction of the changed magnetic field.
Here on the right, the needle has rotated so that with 3 amps of current flowing in the wire underneath the glass dish, the needle now points (almost) at right angles to the wire. The force on the needle is perpendicular to the wire, and also to the field extending out radially from the wire. The direction of the force is around the wire, like the force on the debris around a tornado.
If I could have got hold of a tiny isolated magnetic pole (north, say) like those in my old school physics book and floated this on the water, it would have accelerated in the direction of the field. But I can't. All I can do is get a pair of poles - north and south - in the same needle. The force on the north pole is exactly counterbalanced by the force in the other direction on the south pole. So the needle can't move. All it can do is point in the direction it would like to move.
When I switch off the current (as on the left), the needle swings back to point north again. So the experiment confirms that the wire is generating its own magnetic field, and exerting a force in a direction at two right angles to the direction of current flow, just as the equations in the textbooks predict it should.
Energy in magnetic fields
The most important thing to know about a magnetic field is that it can store energy. Some textbooks even say that a magnetic field is the name given to a region of space in which an inductor can store energy. How? Google reported that 'Your search for "How does a magnetic field store energy?" did not match with any Web results' so if anyone knows, they're not telling.
But maybe the question is meaningless. Maybe a magnetic field is what we see when we store energy "in" it. From this point of view, "a magnetic field" and "a region of space in which energy is stored" are just different labels for the same phenomenon. If you open a new bank account and store money in it, is it meaningful to ask, How, precisely, is the money stored? Isn't a bank account just a distortion in 'financial' space? The distortion only exists as long as the account is open. Close it, withdraw the money, and the distortion vanishes.
When a current starts to flow in a wire, all the little charge carriers are abruptly woken up and ordered to start moving. To inform the rest of the universe that they will soon be somewhere else, they immediately set up a magnetic field around themselves. It grows outwards from the wire, and would eventually reach the edge of the universe.
Once the charge carriers stop moving, they don't need the field any more, and it disappears. Of course, it takes some energy to establish the field in the first place. This energy is borrowed from the power source, stored in the field, and then returned to the circuit afterwards.
You can watch this process happening. When a voltage is applied across an inductor, the current rises steadily instead of jumping up at once to its final value. Some of the available energy from the source is evidently being diverted away from its usual task of pushing current round the circuit, and instead is being hoarded in the growing magnetic field that now cocoons the inductor. And when the power source is disconnected, this energy comes back into the circuit and tries to maintain the current at its previous level. It fails, of course, but in the process it raises the voltage across the inductor abruptly, sometimes to disastrously high levels, during the few moments the energy is available. This effect (thank you, Don) is used to generate the 20 kV sparks needed by your car's engine.
Energy storage in an inductor
Lenz's law says that, if you try to start current flowing in a wire, the current will set up a magnetic field that opposes the growth of current. The universe doesn't like being disturbed, and will try to stop you. It will take more energy than you expect to get the current flowing. This additional energy isn't lost - it is stored, in the magnetic field established by the current. You get it back when the current stops and the field collapses.
There's another way of looking at this. The magnetic field is caused by the current, and the current is changing - it's growing - so the magnetic field is growing too. But just as a current causes a magnetic field, a changing magnetic field causes a current. (That's how electricity is made, in fact.) As the current grows, the magnetic field it generates tries to force a current to flow backwards along the wire, opposing the original current. The result is that the current grows more slowly than it would have done.
Some people find it helpful to think of this as a back e.m.f. opposing the e.m.f. - the "electromotive force", or voltage, as we say in English - that is causing the current. This voltage is proportional to the rate of change of flux, which in turn is proportional to the rate of change of current. When all the field geometry is lumped into one number - the inductance, L - the relationship between the voltage applied to an inductor and the resulting current flow can be expressed very simply as:
Here's an inductor L being charged from a power supply, with all the instantaneous voltages labelled so that I can set up the equations and explore what's happening.
When power is first applied, the inductor refuses to allow any current to flow. It's not obvious that this should happen, but it does. The current really is zero just after switch-on. Thereafter it begins to rise, following an exponential curve towards its final value of V/R. (You can prove this by writing the equation for the current i and doing the integration, as I did when calculating the time constant of a charging capacitor.) There are some nice video demonstrations of the results of this behaviour on the web - try searching for "Lenz's law".
It's now remarkably easy to calculate the energy stored in the inductor's magnetic field.
I can write the equation for the power absorbed by the inductor as the product of the voltage across it and the current flowing through it. Then, since the current i is changing as the magnetic field builds up, I simply integrate all these different values of i from switch-on to the steady state current I = V/R to get the total power. The energy needed to supply this power has been borrowed from the circuit and is now stored in the inductor's magnetic field for as long as the field persists.
Inductance of a wire
Inductance of a long straight wire
For completeness, I include here a way to calculate the inductance of a long, straight wire of length s (mm) and, diameter d (mm):
L (nH) = 0.2 s { ln(4s/d) - 0.75 }
It looks complicated, but in fact it works out at around 1.5 μH for a 1 metre length or 3 mH for a kilometre for most gauges of wire.